Saturday, August 04, 2007
Is Reality a misunderstanding?
April 29, 2007Special to World Science
Several physicists say they’ve confirmed strange predictions of modern physics that clash with our most basic notions of reality and even suggest—some scientists and philosophers say—that reality isn’t
 there when we’re not looking.
there when we’re not looking.The predictions have lurked within quantum mechanics, the science of the smallest things, since the field emerged in the 1920s; but not all physicists accepted them. They were undisputedly consistent with experiments, but experiments might not reveal everything.
New tests—designed more specifically than before to probe the reality question—have yielded unsettling results, say researchers who published the findings in the April 19 issue of the research journal Nature. One of their colleagues called the findings intriguing but inconclusive.
The background
The background
Quantum physicists have long noted that subatomic particles seem to move randomly. For instance, one can measure a particle’s location at a given moment, but can’t know exactly where it would be just before or after.
Physicists determined that the randomness wasn’t just an appearance due to our ignorance of the details of the motion, but an inescapable property of the particles themselves.
Rather persuasive evidence for this lay in math. Particles, for reasons no one quite knows, sometimes act like waves. When they come together, they create the same types of complex patterns that appear when water ripples from different directions overlap.
But a particle, being at least somewhat confined in space, normally acts only as a small “wave packet”—a cluster of a few ripples in succession—unlike familiar waves, in which dozens or thousands parade along.
It turns out there is a mathematical way to represent a wave packet; but you must start by representing an infinitely repeating wave, which is a simpler formula. Adding up many such depictions, if you choose them properly, gives the packet.
Yet there’s a catch: each of these components must have a slightly different wave speed. Thus, the complete packet has no clear-cut speed. Nor, consequently, does the particle.
The previous experiments
Precisely in line with such math, experiments find that particle speed is somewhat random, though the randomness follows rules that again mirror the equations. When you measure speed, you do get a number, but that won’t tell you the speed a moment before or after. In essence, physicists concluded, the particle has no defined velocity until you measure it. Similar considerations turned out to hold for its location, spin and other properties.
The implications were huge: the randomness implied that key properties of these objects, perhaps the objects themselves, might not exist unless we are watching. “No elementary phenomenon is a phenomenon until it is an observed phenomenon,” the celebrated Princeton University physicist John Wheeler put it.
Still, human-made mathematical models don’t necessarily reflect ultimate truth, even if they do match experimental results brilliantly. And those tests themselves might miss something. Scientists including Einstein balked at the randomness idea—“God does not play dice,” he famously fumed—and the consequent collapse of cherished assumptions. The great physicist joined others in proposing that there exist some yet-unknown factors, or “hidden variables,” that influence particle properties, making these look random without truly being so.
Physicists in due course designed experiments to test for hidden variables. In 1964 John Bell devised such a test. He exploited a curious phenomenon called “entanglement,” in which knowing something about one particle sometimes tells you a corresponding property of another, no matter the distance between them.
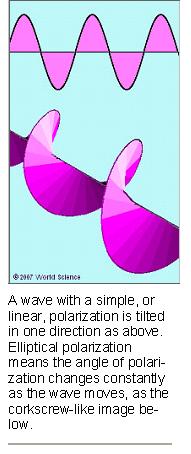
An example occurs when certain particles decay, or break up, into two photons—particles of light. These fly off in opposite directions and have the same polarization, or amount by which the wave is tilted in space. Detectors called polarizers can measure this attribute. Polarizers are like tiny fences with slits. If the slits are tilted the same way as the wave, it goes through; if oppositely, it doesn’t; if somewhere in between, it may or may not pass.
If you measure the two oppositely-flying photons with polarizers tilted the same way, you get the same result for both. But if one of the polarizers is tilted a bit, you will get occasional disagreements between the results.
What if you also tilt the second polarizer by the same amount, but the opposite way? You might get twice as many disagreements, Bell reasoned. But you might also get less than that, because some potential disagreements could cancel each other out. For example: two photons might be blocked whereas originally they both would have passed, so two deviations from the original result lead to an agreement.
All this follows from logic. It also depends on certain reasonable assumptions, including that the particles have a real polarization whether it’s measured or not.
But Bell, in an argument known as Bell’s Theorem, showed that quantum mechanics predicts another outcome, implying this “reality” assumption might be wrong. Quantum mechanics claims that the number of disagreements between the results when both polarizers are oppositely tilted—compared to one being tilted—can be more than twice as many. And experiments have borne this out.
The reasons why have to do with yet another odd prediction of quantum mechanics. Once you detect the photon as either having crossed the polarizer or not, then it’s either polarized exactly in the direction of the instrument, or the opposite way, respectively. It can’t be polarized at any other angle. And its “twin” must be identically polarized. All this puts additional constraints on the system such that the number of disagreements can rise compared to the “logical” result.
Past experiments have confirmed the seemingly nonsensical outcome. Yet this alone this doesn’t disprove the “reality” hypothesis, researchers say. There’s one other possibility, which is that the particles are somehow instantaneously communicating, like telepaths.
The new experiment
The new experiment was designed to sidestep this loophole: it was set up so that even allowing for instantaneous communication couldn’t explain the “nonsensical” outcome, at least not easily. One would also have to drop the notion that photons have a definite polarization independent of any measurement.
The work, by Simon Groeblacher and colleagues at the Austrian Academy of Sciences’ Institute for Quantum Optics and Quantum Information in Vienna, was based not on Bell’s Theorem, but on a related theorem more recently developed by Anthony Leggett at the University of Illinois at Urbana-Champaign.
Full experiments based on Leggett’s concept required analyzing photon-waves that are polarized “elliptically,” which means a wave’s tilt changes constantly. One can detect this by supplementing the polarizer with a strip of material that’s birefringent, meaning it bends light differently depending on its direction.
The results indeed disproved that photons have a definite, independently existing polarization, Markus Aspelmeyer, a member of the research team, wrote in an email. The findings thus spell trouble for one “plausible notion of realism,” he added, though others could conceivably survive.
Not everyone is convinced. “The conclusion one draws is more a question of taste than logic,” wrote Alain Aspect, who conducted the first conclusive tests of Bell’s Theorem, in a commentary in the same issue of the journal. Aspect, of the École Polytechnique in Palaiseau, France, argued that the findings can still be explained by claiming certain forms of instantaneous communication. But he conceded that he too is inclined to renounce aspects of realism instead. Such experiments, and the resulting debates, “allow us to look deeper into the great mysteries of quantum mechanics,” he added
